Ship-generated Loading

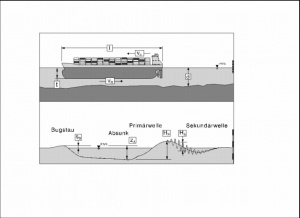
The change in draught- and speed-related ship-induced loading on the shipping routes entails a definition of the characteristic parameters (e.g. ship wave system, displacement current) and requires knowledge of the functional relationships (e.g. ship speed and vessel width, shipping channel conditions, passing clearance). Picture 1 shows a diagram of the ship wave system among others.

Since the start of the new century, a large number of analytical and empirical methods have been developed for calculating the interaction of ship and waterway. Initial calculations with hydrodynamic-numerical models reveal deviations compared to the results of hydraulic scale models, so that these numerical models cannot yet be viewed as a confirmed scientific aid for determining upgrading-related changes to ship-induced loading in shipping channels. At the moment, it is only possible to make confirmed quantitative predictions of ship-induced loadings in inhomogeneous waterways by means of hydraulic model tests in a scientifically confirmed model scale (state-of-the-art engineering and science).

In recent years, the BAW Hamburg office has looked at the issue of the interaction between seagoing ship and tidal fairways both with studies on hydraulic models and with field study measurements.

Definition of the Characteristic Parameters

Graph 1: Parameters and Functional Dependencies

A moving ship generates waves of different periods as a consequence of its displacement current causing pressure and water level changes at its bow, stern and alongside its hull. The following parameters describe the ship wave and current system, for example, for a ship moving in an estuary at subcritical speed (ship speed less than the phase speed of the prevailing waves):

• the bow pressure (s_B) directly at the hull,
• the water level depression (z_A) at the side of the ship,
• the stern wave (H_P) which is a part of the long period primary wave system,
• the secondary wave superimposed on the primary wave system (H_S),
• velocity (v_R) of the simultaneously occurring return current.

The water level changes for a depth- and width-limited waterway, as seen by an observer from the bank, are shown as a vertically exaggerated sideview in the above sketch.

Functional Relationships

The parameters for the water level and current variations caused by a moving ship are a function of:

• ship velocity v_S and the passing distance L
• ship dimensions (length l, width b, draught t, submerged midship cross-section A_S)
• total hydrodynamic resistance of the ship (ship form) in the canal R_T,K
• waterway conditions (water level B and bed width B_S, water depth d, cross-section and area A, bank profile and slope 1 : m)
• current conditions in the waterway
• other influences such as waterway bend, type of propulsion, water density.

The essential parameters for the ship induced loading for fairways have been shown to be:

• the ship velocity (v_S)
• the passing distance (L) to the bank edge which defines the hydraulic partial cross-section (A_T)
• the water depth to draught ratio (d/t), and the cross-section ratio A_T / 0,5 A_S.

To illustrate more simply the physical processes occurring when a ship is moving in a waterway of varying non-uniform cross-section, one may think of the waterway cross-section as being divided into two parts A_T1 and A_T2, through each of which half of the ship's displacement passes. Thus each bank experiences a qualitatively different ship induced loading as is determined by the partial cross-section ratios A_T1 / 0,5 A_S and A_T2 / 0,5 A_S.

Analytical and Empirical Methods

Traditional Methods

Graph 1: spectrum of possible calculated results

Whereas analytical derivations can be used for the calculation of the water level depression z_A (e.g. KREY, 1913; CONSTANTINE, 1960; BOUWMEESTER et al., 1977; FÜHRBÖTER, 1982), the calculation methods for determining the wave heights of ship waves, often aided by model studies and/or field measurements, are empirical (e.g., RÖMISCH, 1969). Though the short period secondary waves are taken into account by some of the empirical approaches, they are not known in detail as functions of the described parameters. This is because these secondary waves are generated as a function of ship speed and ship form and as a result of the different pressure distributions along the body of the ship.

The following simplified relationships have been culled from the literature for the subcritical speed range (i.e., v_S < 0.9·[g·d]^0.5).This is the range used, for economical reasons, by merchant shipping:

• water level depression and wave height
  z_A proportional to v_S^k with 2 < k < 3.5
  z_A proportional to n^k with -1.5 < k < -1

• return current velocity
  v_R proportional to v_S
  v_R proportional to n^-1

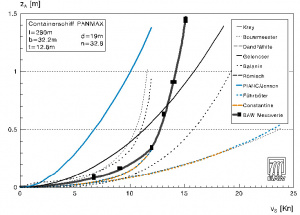
The diagram (Graph 1) shows the spectrum of possible calculated results given the boundary conditions at a cross-section of the Lower Elbe compared to the measurement data won from a hydraulic model of the BAW-DH.

Not only is the interaction between ship and waterway critical for forming a prognosis on ship induced loading in waterways with varying non-uniform cross-sections, but also the physical processes of wave spreading such as refraction and shoaling are critical and must be accounted for in the calculations (without parameterization).

Traditional empirical and analytical approaches only allow for an inadequate estimation of the ship induced loading caused by sea-going merchant shipping on large fairways with varying non-uniform cross-sectional geometries, especially in tidal regions. Thus these approaches are inadequate for calculating the magnitude of future loading on waterways.

Hydrodynamic Numerical Methods

Author: K. Uliczka

• Definition of the Characteristic Parameters
• Functional Relationships
• Analytical and Empirical Methods
• Hydrodynamic Numerical Methods

---

back to Hydraulic Modelling

---

Overview